The length of UC is 18 units.
To find the length of UC, we need to use the given information and equations involving other lengths. Let's break down the solution step by step:
Step 1:
Find the length of HK by first establishing the equation for JK:
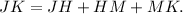
Given that

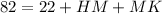
Subtract 22 from both sides to isolate the unknowns:
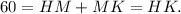
Step 2:
Incorporate HK into the equation involving KU:

Substituting
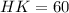
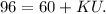
Subtract 60 from both sides to isolate the unknown:
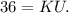
Step 3:
Utilize KU and solve for UC in the final equation:
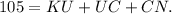
Given that
 , and
, and
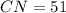
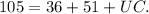

Subtract 87 from both sides to isolate the unknown:
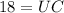
Therefor, the length of UC is 18 units.