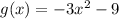Answer:
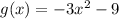
Step-by-step explanation:
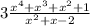
+
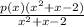
We need p(x) need to be a degree 2 polynomial so the numerator of the second fraction is degree 4. Our goal is to cancel the terms of the first fraction's numerator that are of degree 2 or higher.
So let p(x)=ax^2+bx+c.
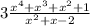
+
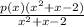
Plug in our p:
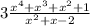
+
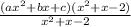
Take a break to multiply the factors of our second fraction's numerator.
Multiply:
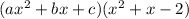
=
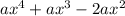
+
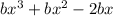
+
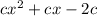
=
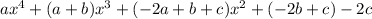
Let's go back to the problem:
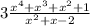
+
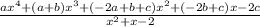
Let's distribute that 3:
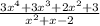
+
![(ax^4+(a+b)x^3+(-2a+b+c)x^2+(-2b+c)x-2c)/(x^2+x-2)[/tex</p><p></p><p>So this forces [tex]a=-3](https://img.qammunity.org/2022/formulas/mathematics/high-school/xbrpiyii1ja77127bq4qlzfqrx2qdp1ywj.png) .
.
Next we have
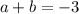 . Based on previous statement this forces
. Based on previous statement this forces
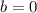 .
.
Next we have
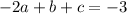 . With
. With
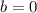 and
and
 , this gives
, this gives
 .
.
So
![c=-9[tex].</p><p></p><p>Next we have the x term which we don't care about zeroing out, but we have [tex]-2b+c]() which equals
which equals
 .
.
Lastly,
 .
.
This makes
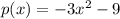 .
.
This implies
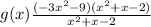 or simplified
or simplified