9514 1404 393
Answer:
1 23/24
Explanation:
Fractions can be added when they have the same denominator. Then the addition is performed by adding the numerators, and expressing the sum over the common denominator.
Here, your fractions have denominators of 8 and 6. Usually, we want to find a "least common denominator" to use to express the fractions. There are various ways to find that value. One of the easiest is to consult your memory of multiplication tables to find the smallest number that both a multiple of 8 and a multiple of 6. That number is 24.
An equivalent fraction is one that has the same value, but a different denominator than the one it is being compared to. Equivalent fractions can be made by multiplying by "1" in the form of "a/a" where "a" is any non-zero value. Here, it is useful to multiply 9/8 by 3/3 to make the equivalent fraction 27/24, which has a denominator of 24.
Similarly, we can multiply 5/6 by 4/4 to get the equivalent 20/24, which also has a denominator of 24.
These two fractions can now be added:
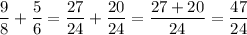
If you want to turn this into a "mixed number", you need to find how many times 24 goes into 47: 47÷24 = 1 remainder 23. The quotient is the integer part of the mixed number; the remainder is the numerator of the fractional part. Then the mixed number value of the sum is ...
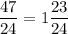
_____
Additional comments
The product of the denominators can always serve as a common denominator. That may not be the "least" common denominator. If you use that here, you would have ...

This result can be reduced by removing a factor of 2 from numerator and denominator to give 47/24, the sum we had above.
The "least common denominator" (LCD) is the Least Common Multiple (LCM) of the denominators. It can be found by forming the product of the unique factors of the denominators. Here, we have 8 = 2·2·2 and 6 = 2·3. The LCD is the product 2·2·2·3. We recognize that 2³ and 3 are unique factors that need to contribute to the LCD. 2 is subsumed by 2³.
As you can see from the factoring, 2 is a common factor of both numbers. Another way to find the LCD (or LCM of the denominators) is to form their product (8×6 = 48) and divide that by the greatest common factor (GCF), which is 2. (48/2 = 24, the LCD) Sometimes it is easier to find the GCF and compute (product/GCF) than to find the LCM using factoring.
__
If you don't mind the possibility of having to reduce the resulting fraction, the sum of fractions can always be computed as ...
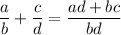
This formula computes 94/48 as the sum of these fractions, effectively leaving out the middle step (9/8×6/6 +...) shown in the work above. I find this especially useful for adding rational expressions, not just numerical fractions.