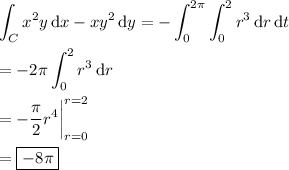It looks like the integral you want to find is

where C is the circle x ² + y ² = 4. By Green's theorem, the line integral is equivalent to a double integral over the disk x ² + y ² ≤ 4, namely
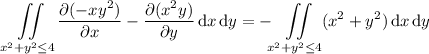
To compute the remaining integral, convert to polar coordinates. We take
x = r cos(t )
y = r sin(t )
x ² + y ² = r ²
dx dy = r dr dt
Then