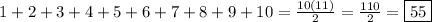Answer:
Explanation:
We'll be using case-work to solve this problem. Let's call the ones digit of the telephone number
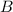 and the tens digit
and the tens digit
 . Each telephone number can be represented as
. Each telephone number can be represented as
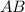 .
.
Since the question states that numbers that are smaller when their digits are reversed are not used, we have the following inequality:
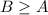
This is because if
 , the number would become smaller when
, the number would become smaller when
 and
and
 are switched in
are switched in
 . However, if
. However, if
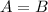 or
or
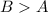 , the number will not become smaller.
, the number will not become smaller.
Let's work our way up starting with
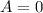 . If
. If
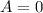 , there are 10 other numbers (0-9) that we can choose for
, there are 10 other numbers (0-9) that we can choose for
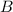 that adhere to the condition
that adhere to the condition
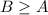 :
:
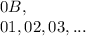
Therefore, there are 10 possible telephone numbers when the tens digit is 0.
Repeat the process, now assigning
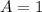 . Now, we only have the digits 1-9 to choose from for
. Now, we only have the digits 1-9 to choose from for
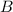 , since
, since
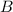 needs to be greater than or equal to A. Therefore, there are 9 possible telephone numbers when the tens digit is 1.
needs to be greater than or equal to A. Therefore, there are 9 possible telephone numbers when the tens digit is 1.
This pattern continues. As we work our way up through the cases (when increasing
 by 1), the number of possible telephone numbers decreases by 1, since there becomes one less option for
by 1), the number of possible telephone numbers decreases by 1, since there becomes one less option for
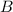 .
.
The last case would be
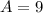 in which case there would only be one option for
in which case there would only be one option for
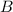 and that would be 9.
and that would be 9.
Since there are 10 cases (0-9), add up the possible telephone numbers for each case:

Alternatively, recall that the sum of this series can be found using
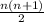 , where
, where
 is the number of values in the set. In this case,
is the number of values in the set. In this case,
 , and we have:
, and we have: