Answer:
The p-value of the test is 0.0023 < 0.02, which means that there is sufficient evidence at the 0.02 level to dispute the company's claim.
Explanation:
The company's promotional literature claimed that 28% do not fail in the first 1000 hours of their use.
At the null hypothesis, we test that at least 28% do not fail, that is:
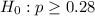
At the alternative hypothesis, we test if the proportion is of less than 28%, that is:
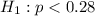
The test statistic is:

In which X is the sample mean,
 is the value tested at the null hypothesis,
is the value tested at the null hypothesis,
 is the standard deviation and n is the size of the sample.
is the standard deviation and n is the size of the sample.
0.28 is tested at the null hypothesis:
This means that

Sample of 1800 computer chips revealed that 25% of the chips do not fail in the first 1000 hours of their use.
This means that

Value of the test statistic:

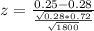
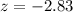
P-value of the test and decision:
The p-value of the test is the probability of finding a sample proportion below 0.25, which is the p-value of Z = -2.83.
Looking at the z-table, z = -2.83 has a p-value of 0.0023.
The p-value of the test is 0.0023 < 0.02, which means that there is sufficient evidence at the 0.02 level to dispute the company's claim.