Answer:
a) The sample mean is 1260 and the standard deviation is 48.
b) The 90% confidence interval for the mean of all tree-ring dates from this archaeological site is (1230, 1290).
Explanation:
Question a:
Mean is the sum of all values divided by the number of values. So
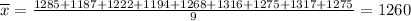
Standard deviation is the square root of the sum of the differences squared between each value and the mean, divided by the one less than the sample size. So
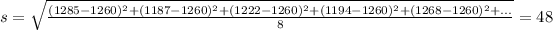
The sample mean is 1260 and the standard deviation is 48.
Question b:
We have the standard deviation for the sample, which means that the t-distribution is used to solve this question.
The first step to solve this problem is finding how many degrees of freedom,which is the sample size subtracted by 1. So
df = 9 - 1 = 8
90% confidence interval
Now, we have to find a value of T, which is found looking at the t table, with 8 degrees of freedom(y-axis) and a confidence level of
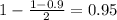 . So we have T = 1.8595
. So we have T = 1.8595
The margin of error is:
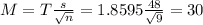
In which s is the standard deviation of the sample and n is the size of the sample.
The lower end of the interval is the sample mean subtracted by M. So it is 1260 - 30 = 1230
The upper end of the interval is the sample mean added to M. So it is 1260 + 30 = 1290
The 90% confidence interval for the mean of all tree-ring dates from this archaeological site is (1230, 1290).