Compute the quotient and remainder,
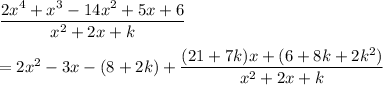
The remainder upon dividing
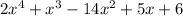 by
by
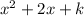 should leave no remainder, which means
should leave no remainder, which means
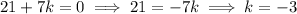
and
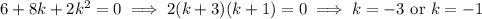
Only k = -3 makes both remainder terms vanish.
Then the previous result reduces to
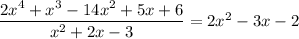
so that
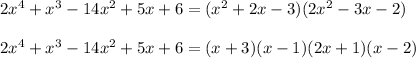
and so the zeroes of the quartic polynomial are x = -3, x = 1, x = -1/2, and x = 2.