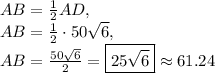Question

Looking at the top of tower A and base of tower B from points C and D, we find that ∠ACD = 60°, ∠ADC = 75° and ∠ADB = 30°. Let the distance between points C and D be 100. Find the height AB of the tower.

Picture attached for the problem. Please show your work too. Thanks!

Answer 1

`\text{Exact: }AB=25√(6),\\\text{Rounded: }AB\approx 61.24`

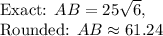
Explanation:

We can use the Law of Sines to find segment AD, which happens to be a leg of
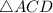`\triangle ACD` and the hypotenuse of
`\triangle ADB`.

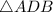The Law of Sines states that the ratio of any angle of a triangle and its opposite side is maintained through the triangle:

`(a)/(\sin \alpha)=(b)/(\sin \beta)=(c)/(\sin \gamma)`

Since we're given the length of CD, we want to find the measure of the angle opposite to CD, which is
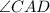`\angle CAD`. The sum of the interior angles in a triangle is equal to 180 degrees. Thus, we have:

`\angle CAD+\angle ACD+\angle CDA=180^(\circ),\\\angle CAD+60^(\circ)+75^(\circ)=180^(\circ),\\\angle CAD=180^(\circ)-75^(\circ)-60^(\circ),\\\angle CAD=45^(\circ)`

Now use this value in the Law of Sines to find AD:

`(AD)/(\sin 60^(\circ))=(100)/(\sin 45^(\circ)),\\\\AD=\sin 60^(\circ)\cdot (100)/(\sin 45^(\circ))`

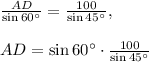
Recall that
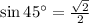`\sin 45^(\circ)=(√(2))/(2)` and
`\sin 60^(\circ)=(√(3))/(2)`:

`AD=((√(3))/(2)\cdot 100)/((√(2))/(2)),\\\\AD=(50√(3))/((√(2))/(2)),\\\\AD=50√(3)\cdot (2)/(√(2)),\\\\AD=(100√(3))/(√(2))\cdot( √(2))/(√(2))=(100√(6))/(2)={50√(6)}`

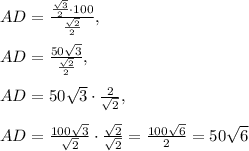
Now that we have the length of AD, we can find the length of AB. The right triangle
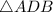`\triangle ADB` is a 30-60-90 triangle. In all 30-60-90 triangles, the side lengths are in the ratio
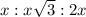`x:x√(3):2x`, where
`x` is the side opposite to the 30 degree angle and
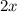`2x` is the length of the hypotenuse.

Since AD is the hypotenuse, it must represent
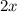`2x` in this ratio and since AB is the side opposite to the 30 degree angle, it must represent
`x` in this ratio (Derive from basic trig for a right triangle and
`\sin 30^(\circ)=(1)/(2)`).

Therefore, AB must be exactly half of AD:

`AB=(1)/(2)AD,\\AB=(1)/(2)\cdot 50√(6),\\AB=(50√(6))/(2)=\boxed{25√(6)}\approx 61.24`

Answer 2

`\displaystyle 25 √(6)`

Explanation:

the triangle ∆ABD is a special right angle triangle of which we want to figure out length of its shorter leg (AB).

to do so we need to find the length of AD (the hypotenuse). With the help of ∆ADC it can be done. so recall law of sin

`\boxed{ \displaystyle ( \alpha )/( \sin( \alpha ) ) = ( \beta )/( \sin( \beta ) ) = ( c)/( \sin( \gamma ) ) }`

we'll ignore B/sinB as our work will be done using the first two

step-1: assign variables:

• 
  `\sin( \gamma ) = \sin( {60}^( \circ) )`
• 
  `c=AD`
• 
  `\rm \sin( \alpha ) = \sin( {180}^( \circ) - ({60}^( \circ) + {75}^( \circ) )) = \sin( {45}^( \circ) )`
• 
  `a=100`

step-2: substitute

`\displaystyle \frac{100}{ \sin( {45}^( \circ) )} = \frac{AD }{ \sin( {60}^( \circ) )}`

recall unit circle therefore:

`\displaystyle (100)/( ( √(2) )/(2) ) = (AD )/( ( √(3) )/(2) )`

simplify:

`AD = 50 √(6)`

since ∆ABD is a 30-60-90 right angle triangle of which the hypotenuse is twice as much as the shorter leg thus:

`\displaystyle AB = (50 √(6) )/(2)`

simplify division:

`\displaystyle AB = \boxed{25 √(6) }`

and we're done!