Answer:
The answer is
 .
.
Explanation:
To solve this problem, start by factoring the equation using the perfect square trinomial rule, which is states that the middle term is the first term multiplied by the last term, and then multiplied by 2. The formula for the perfect square trinomial rule looks like
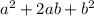 , where
, where
 and
and
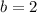 . The equation will look like
. The equation will look like
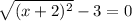 .
.
Next, pull out the terms from under the radical, assuming positive real numbers, which will look like
 . Then, simplify the equation by subtracting 3 from 2, which will look like
. Then, simplify the equation by subtracting 3 from 2, which will look like
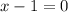 . Finally, add 1 to both sides of the equation, and the answer will be
. Finally, add 1 to both sides of the equation, and the answer will be
 .
.