Answer:
New pressure, P2 = 204.45 kPa
Step-by-step explanation:
Given the following data;
Initial temperature, T1 = -10°C
Initial pressure, P1 = 190 kPa
Final temperature, T2 = 10°C
First of all, we would have to convert the value of the temperatures in Celsius to Kelvin.
Conversion:
Kelvin = 273 + °C
For T1;
Kelvin = 273 + (-10)
Kelvin 273 - 10 = 263 K
For T2;
Kelvin = 273 + 10 = 283 K
To find the new pressure, we would use Gay Lussac's law;
Gay Lussac states that when the volume of an ideal gas is kept constant, the pressure of the gas is directly proportional to the absolute temperature of the gas.
Mathematically, Gay Lussac's law is given by;


Making P2 as the subject formula, we have;
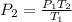
Substituting the values into the formula, we have;
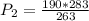
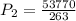
New pressure, P2 = 204.45 kPa