I think the given series is
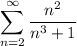
You can use the integral test because the summand is clearly positive and decreasing. Then
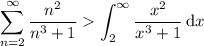
Substitute u = x ³ + 1 and du = 3x ² dx, so the integral becomes
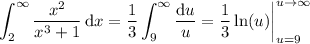
As u approaches infinity, we have ln(u) also approaching infinity (whereas 1/3 ln(9) is finite), so the integral and hence the sum diverges.