Answer:
0.25 = 25% probability that the two brothers will end up in the starting line up
Explanation:
A probability is the number of desired outcomes divided by the number of total outcomes.
The order in which the players are chosen is not important, which means that the combinations formula is used to solve this question.
Combinations formula:
 is the number of different combinations of x objects from a set of n elements, given by the following formula.
is the number of different combinations of x objects from a set of n elements, given by the following formula.

Desired outcomes:
Matthew plus another forward from a set of 3.
Tony plus another two guards from a set of 5.
So

Total outcomes:
Two forwards from a set of 4.
Three guards from a set of 6.
So
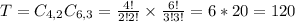
What is the probability that the two brothers will end up in the starting line up?

0.25 = 25% probability that the two brothers will end up in the starting line up