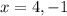Answer: x=4, -1
Step-by-step explanation:
Assuming you meant
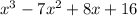 , the zeros of the question are x = 4 and -1.
, the zeros of the question are x = 4 and -1.
Step 1. Replace f(x) with y.
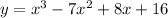
Step 2. To find the roots of the equation, replace y with 0 and solve.
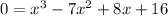
Step 3. Factor the left side of the equation.
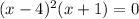
Step 4. Set x-4 equal to 0 and solve for x.
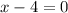
Step 5. Set
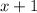 equal to 0 and solve for x.
equal to 0 and solve for x.
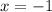
The solution is the result of
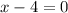 and
and
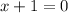 .
.