Question

Suppose that, in the past, 40% of all adults favored capital punishment. Do we have reason to believe that the proportion of adults favoring capital punishment today has increased if, in a random sample of 15 adults, 8 favor capital punishment? Use a 0.05 level of significance.

Answer 1

The p-value of the test is 0.1469 > 0.05, which means that there is no reason to believe that the proportion of adults favoring capital punishment today has increased, using a 0.05 level of significance.

Explanation:

Suppose that, in the past, 40% of all adults favored capital punishment. Test if the proportion has increased:

At the null hypothesis, we test if the proportion is still of 40%, that is:

`H_0: p = 0.4`

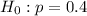
At the alternative hypothesis, we test if the proportion has increased, that is, is greater than 40%, so:

`H_1: p > 0.4`

The test statistic is:

`z = (X - \mu)/((\sigma)/(√(n)))`

In which X is the sample mean,
`\mu` is the value tested at the null hypothesis,
`\sigma` is the standard deviation and n is the size of the sample.

0.4 is tested at the null hypothesis:

This means that
`\mu = 0.4, \sigma = √(0.4*0.6)`

Random sample of 15 adults, 8 favor capital punishment.

This means that
`n = 15, X = (8)/(15) = 0.5333`

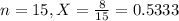
Value of the test statistic:

`z = (X - \mu)/((\sigma)/(√(n)))`

`z = (0.5333 - 0.4)/((√(0.4*0.6))/(√(15)))`

`z = 1.05`

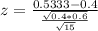
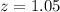
P-value of the test and decision:

The p-value of the test is the probability of finding a sample proportion of 0.5333 or more, which is 1 subtracted by the p-value of z = 1.05.

Looking at the z-table, z = 1.05 has a p-value of 0.8531.

1 - 0.8531 = 0.1469.

The p-value of the test is 0.1469 > 0.05, which means that there is no reason to believe that the proportion of adults favoring capital punishment today has increased, using a 0.05 level of significance.