Question

To all the physicians please help this is for my assignment

Answer 1

Q. 1. Newton's Law of gravitation states that all bodies in the universe exerts a force of attraction on all other bodies in the universe with a proportional force to both the product of the masses of the bodies and inversely proportional to the square of the distance between their centers

Mathematically, we have;

`F = G * (m_1 * m_2)/(R^2)`

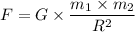
Where;

m₁, and m₂ are the masses of the bodies

R = The distance between their centers

G = The gravitational constant = 6.6743 × 10⁻¹¹ N·m²/kg²

The gravitational constant, G, is the Newton's law of gravitation's constant of proportionality between the force of attraction that exist two bodies and the product of their masses divided by the square of the distance between their centers

Q. 2. Newton's law of gravitation in vector form is presented as follows;

`\underset{F_(12)}{\rightarrow} = -G * (m_1 * m_2)/(R_(21)^2) \cdot \hat R_(12)`

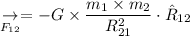
The above equation gives the gravitational force of attraction of body 1 on body 2, with the negative sign and unit vector indicating that the force of of gravity is towards body 1

The force of gravity of body 2 on 1 is presented as follows;

`\underset{F_(12)}{\rightarrow} = -G * (m_1 * m_2)/(R_(12)^2) \cdot \hat R_(21)`

The gravitational force of attraction of body 2 on body 1 is therefore, equal in magnitude and opposite in direction of the gravitational force of body 1 on body 2 (towards body 2)

`-\underset{F_(12)}{\rightarrow} = G * (m_1 * m_2)/(R_(21)^2) \cdot \hat R_(12) = G * (m_1 * m_2)/(R_(21)^2) \cdot -(\hat R_(21)) = -G * (m_1 * m_2)/(R_(21)^2) \cdot \hat R_(21)`

`-\underset{F_(12)}{\rightarrow} = -G * (m_1 * m_2)/(R_(21)^2) \cdot \hat R_(21) = \underset{F_(21)}{\rightarrow}`

`-\underset{F_(12)}{\rightarrow} = \underset{F_(21)}{\rightarrow}`

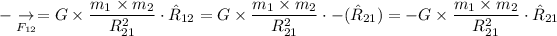
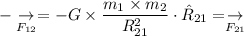
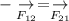
Step-by-step explanation: