Answer:
0.001687 = 0.1687% probability that no more than 1 vessel transporting nuclear weapons was destroyed.
Explanation:
The vessels are destroyed and then not replaced, which means that the hypergeometric distribution is used to solve this question.
Hypergeometric distribution:
The probability of x successes is given by the following formula:
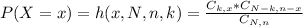
In which:
x is the number of successes.
N is the size of the population.
n is the size of the sample.
k is the total number of desired outcomes.
Combinations formula:
 is the number of different combinations of x objects from a set of n elements, given by the following formula.
is the number of different combinations of x objects from a set of n elements, given by the following formula.

In this question:
Fleet of 18 means that
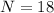
9 are carrying nuclear weapons, which means that
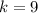
9 are destroyed, which means that
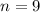
What is the probability that no more than 1 vessel transporting nuclear weapons was destroyed?
This is:
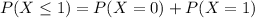
In which
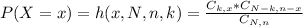
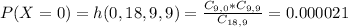
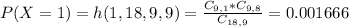
Then
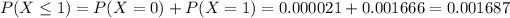
0.001687 = 0.1687% probability that no more than 1 vessel transporting nuclear weapons was destroyed.