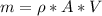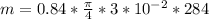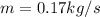Answer:
a)
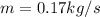
b)

Step-by-step explanation:
From the question we are told that:
Pressure
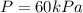
Diameter
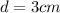
Generally at sea level
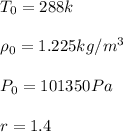
Generally the Power series equation for Mach number is mathematically given by
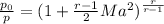
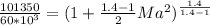

Therefore
Mass flow rate
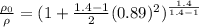
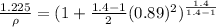
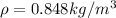
Generally the equation for Velocity at throat is mathematically given by
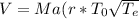 )
)
Where

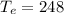
Therefore
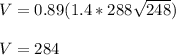
Generally the equation for Mass flow rate is mathematically given by