Question

PLEASE HELP ASAP

A helicopter hovers 500 feet
above a small island. The
figure shows that the angle of
depression from the helicopter
to point P is 48º. How far off
the coast, to the nearest foot, is
the island?
d ft
(Round the answer to the nearest whole number.)

Answer 1

d = 450 ft

Explanation:

Hi there!

First, we can find the angle in the triangle at the point of the helicopter, the angle opposite
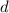`d`. Subtract 48° from 90°:

90-48=42

Now, given that this is a right triangle and we have an angle and the side adjacent the angle, we can solve for
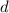`d` by using the tangent ratio:

`tan\theta=\displaystyle (opposite)/(adjacent)`

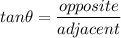
Replace θ with 42°, "opposite" with
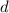`d`, and "adjacent" with 500 ft:

`tan(42)=\displaystyle (d)/(500)`

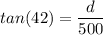
Multiply both sides by 500 to isolate
`d`:

`500*tan(42)=d\\450=d`

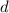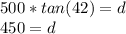
Therefore, d = 450 ft when rounded to the nearest whole number.

I hope this helps!