Question

4. A solution of 60% fertilizer is to be mixed with a solution of 21% fertilizer to form 234 liters of a 43% solution. How many liters of the 60% solution must be used?

SHOW YOUR WORK

Answer 1

Given:

A solution of 60% fertilizer is to be mixed with a solution of 21% fertilizer to form 234 liters of a 43% solution.

To find:

The quantity of the 60% solution in the mixture.

Solution:

Let x be the quantity of the 60% solution and y be the quantity of the 21% solution.

Quantity of mixture is 234. So,

`x+y=234`

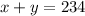
`x=234-y` ...(i)

The mixture has 43% fertilizer. So,

`(60)/(100)x+(21)/(100)y=(43)/(100)* 234`

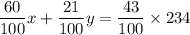
Multiply both sides by 100.

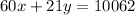`60x+21y=10062` ...(ii)

Using (i) and (ii), we get

`60(234-y)+21y=10062`

`14040-60y+21y=10062`

`-39y=10062-14040`

`-39y=-3978`

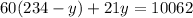
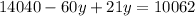
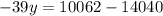
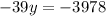
Divide both sides by -39.

`(-39y)/(-39)=(-3978)/(-39)`

`y=102`

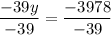
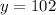
Putting this value in (i), we get

`x=234-102`

`x=132`

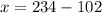
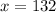
Therefore, 132 liters of the 60% solution must be used.