Question

...what are consecutive multiples ​

Answer 1

H

Explanation:

Let represent A and B

A and B is the sum of the first 50 consecutive multiples of 3 and 6, this is the same as a arithmetic sequence because arithmetic sequences have a common sum.

We can represent this as

`a _(n) = a {}^(1) + d(n - 1)`

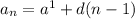
where a^1 is the first term, d is the common sum, n is the number of multiples we adding.

The first term for both A and B respectively is 3 and 6.

Multiples implies that the Difference between A and B are 3 and 6 respectively. There are 50 consecutively. multiples.

Plug in the known parts for both.

`3 + 3(49) = 150`

`6 + 6(49) = 300`

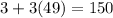
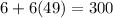
We need to what percent of find 150% of 300.

B is twice as A, so this means that we need to multiply 2 by it original or 100% of it self.

`2 * 100 = 200`

So 200% is the answer.