Answer:
7 and 3
Explanation:
Using the given information, one can form an equation. Let the first number be (x) and the second (y). For two equations based on the given information.
"the sum of two numbers is 10"
x + y = 10
" the sum of their squares is 58 determine"
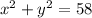
Solve for the equation for (x), then substitute the expression for (x) into the second equation. Simplify and solve to find the value of (y), and backsolve for the value of (x).
x + y =10
x = 10 -y
Substitute into the other equation:
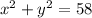
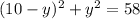
Simplify, remember the pattern for opening a binomial squared:
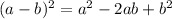
Apply this pattern here to simplify,
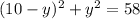
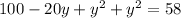
Simplify further,
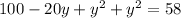
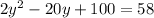
Inverse operations,
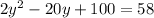
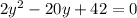
Factor, take out a common factor that all of the terms have. Then rewrite the quadratic trinomial as the product of two linear binomials. Remember the general pattern for factoring, add the inverse of the factors of the constant term to the variable, the sum of these factors must equal the coefficient of the linear term of the quadratic trinomial.
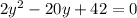
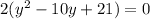

Now use the zero product property to solve, the zero product property states that any number times zero equals zero.

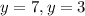
Since the sum of (7) and (3) is (10), one has found the values of (y), it can be either (7) or (3), it doesn't matter which equals which.