SOLUTION :
Given that :

To find :
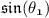
We know that cos θ is base/hypotentuse
So, here the base is -13 and the hypotentuse is 15
As we got the base and hypotentuse, perpendicular needs to be found out
Now, applying Pythagoras Theorem
According to Pythagoras theorem we know that :
- (Hypotentuse)² = (Base)² + (Perpendicular)²
Let us assume perpendicular be x
Putting the values we get
By transposing we get
sin θ formula : Perpendicular/Hypotentuse

Hence, the answer is -2√14/15