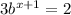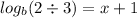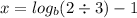Answer:
B the range, the x- and y-intercept
Explanation:
the domain stays the same : all values of x are possible out of the interval (-infinity, +infinity).
but the range changes, as for the original function y could only have positive values - even for negative x.
the new function has a first term (with b) that can get very small for negative x, and then a subtraction of 2 makes the result negative.
the y-intercept (x=0) of the original function is simply y=1, as b⁰=1.
the y-intercept of the new function is definitely different, because the first term 3×(b¹) is larger than 3, because b is larger than 1. and a subtraction of 2 leads to a result larger than 1, which is different to 1.
the original function has no x-intercept (y=0), as this would happen only for x = -infinity. and that is not a valid value.
the new function has an x-intercept, because the y-values (range) go from negative to positive numbers. any continuous function like this must therefore have an x-intercept (again, y = the function result = 0)