Answer:
a) v = 0.4799 m / s, b) K₀ = 1600.92 J, K_f = 5.46 J
Step-by-step explanation:
a) How the two players collide this is a momentum conservation exercise. Let's define a system formed by the two players, so that the forces during the collision are internal and also the system is isolated, so the moment is conserved.
Initial instant. Before the crash
p₀ = m v₁ + M v₂
where m = 95 kg and his velocity is v₁ = -3.75 m / s, the other player's data is M = 111 kg with velocity v₂ = 4.10 m / s, we have selected the direction of this player as positive
Final moment. After the crash
p_f = (m + M) v
as the system is isolated, the moment is preserved
p₀ = p_f
m v₁ + M v₂ = (m + M) v
v =
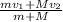
let's calculate
v =
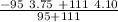
v = 0.4799 m / s
b) let's find the initial kinetic energy of the system
K₀ = ½ m v1 ^ 2 + ½ M v2 ^ 2
K₀ = ½ 95 3.75 ^ 2 + ½ 111 4.10 ^ 2
K₀ = 1600.92 J
the final kinetic energy
K_f = ½ (m + M) v ^ 2
k_f = ½ (95 + 111) 0.4799 ^ 2
K_f = 5.46 J