Answer:
Explanation:
The decay rate of strontium-90 is -.0244 as given.
For b., we have to use the formula to find out how much is left after 30 years. This will be important for part d.
 which simplifies a bit to
which simplifies a bit to
A(t) = 400(.4809461353) so
A(t) = 192.4 g
For c., we have to find out how long it takes for the initial amount of 400 g to decay to 100:
 . Begin by dividing both sides by 400:
. Begin by dividing both sides by 400:
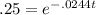 and then take the natural log of both sides:
and then take the natural log of both sides:
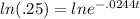 . The natural log and the e cancel each other out since they are inverses of one another, leaving us with:
. The natural log and the e cancel each other out since they are inverses of one another, leaving us with:
ln(.25) = -.0244t and divide by -.0244:
61.8 years = t
For d., we figured in b that after 30 years, 192.4 g of the element was left, so we can use that to solve for the half-life in a different formula:
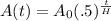 and we are solving for H. Filling in:
and we are solving for H. Filling in:
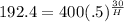 and begin by dividing both sides by 400:
and begin by dividing both sides by 400:
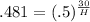 and take the natural log of both sides, which allows us to pull the exponent out front. I'm going to include that step in with this one:
and take the natural log of both sides, which allows us to pull the exponent out front. I'm going to include that step in with this one:
ln(.481) =
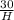 ln(.5) and then divide both sides by ln(.5):
ln(.5) and then divide both sides by ln(.5):
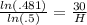 and cross multiply and isolate the H to get:
and cross multiply and isolate the H to get:
 and
and
H = 28.4 years