Given:
Length of ladder = 4.5 meters
Length between ladder and wall = 1.2 meter
To find:
If the angle of the ladder is between 74° - 76°
Steps:
We know,
cos(x) =

Here 'x' is the angle of the ladder. Substituting the values,

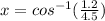
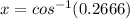
Now we can either use natural cos table or use aa calculator, i am going to use the calculator but if you want to know how to do it with the table, pls comment to this answer.
x = 74.533°
Since x > 74° and x < 76°, it is safe for work
Therefore, the angle is in the safe position for Bryan to use
Happy to help :)
If u need any help, feel free to ask