Question

I operate a small convenience store. Typically, from 10 am to 11 am, I get 23 customers. What is the standard deviation of the number of customers that might check out between 10.15 am and 10.30 am

Answer 1

The standard deviation of the number of customers that might check out between 10.15 am and 10.30 am is 2.4.

Explanation:

We have the mean during a time interval, so the Poisson distribution is used.

Poisson distribution:

In the Poisson distribution, the standard deviation is the square root of the mean.

Typically, from 10 am to 11 am, I get 23 customers.

In an hour, you get 23 customers.

What is the standard deviation of the number of customers that might check out between 10.15 am and 10.30 am?

In this period, there are 15 minutes, that is, one fourth of an hour, so:

`\mu = (23)/(4) = 5.75`

And the standard deviation will be the square root of the mean, so:

`\sigma = √(5.75) = 2.4`

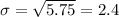
The standard deviation of the number of customers that might check out between 10.15 am and 10.30 am is 2.4.