Solution :
Let
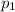 and
and
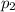 represents the proportions of the seeds which germinate among the seeds planted in the soil containing
represents the proportions of the seeds which germinate among the seeds planted in the soil containing
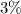 and
and
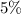 mushroom compost by weight respectively.
mushroom compost by weight respectively.
To test the null hypothesis
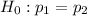 against the alternate hypothesis
against the alternate hypothesis
 .
.
Let
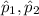 denotes the respective sample proportions and the
denotes the respective sample proportions and the
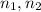 represents the sample size respectively.
represents the sample size respectively.
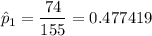
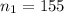
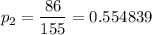
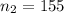
The test statistic can be written as :

which under
 follows the standard normal distribution.
follows the standard normal distribution.
We reject
 at
at
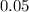 level of significance, if the P-value
level of significance, if the P-value
 or if
or if
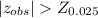
Now, the value of the test statistics = -1.368928
The critical value =
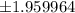
P-value =
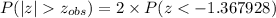
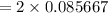
= 0.171335
Since the p-value > 0.05 and
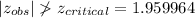 , so we fail to reject
, so we fail to reject
 at
at
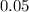 level of significance.
level of significance.
Hence we conclude that the two population proportion are not significantly different.
Conclusion :
There is not sufficient evidence to conclude that the
 of the seeds that
of the seeds that
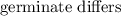 with the percent of the
with the percent of the
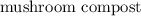 in the soil.
in the soil.