Question

a test for diabetes results in a positive test in 95% of the cases where the disease is present and a negative test in 07% of the cases where the disease is absent. if 10% of the population has diabetes, what is the probability that a randomly selected person has diabetes, given that his test is positive

Answer 1

0.9378 = 93.78% probability that a randomly selected person has diabetes, given that his test is positive.

Explanation:

Conditional Probability

We use the conditional probability formula to solve this question. It is

`P(B|A) = (P(A \cap B))/(P(A))`

In which

P(B|A) is the probability of event B happening, given that A happened.

`P(A \cap B)` is the probability of both A and B happening.

P(A) is the probability of A happening.

In this question:

Event A: Positive test

Event B: Person has diabetes.

Probability of a positive test:

0.95 out of 0.1(person has diabetes).

0.007 out of 1 - 0.1 = 0.9(person does not has diabetes). So

`P(A) = 0.95*0.1 + 0.007*0.9 = 0.1013`

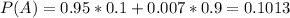
Probability of a positive test and having diabetes:

0.95 out of 0.1. So

`P(A \cap B) = 0.95*0.1 = 0.095`

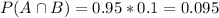
What is the probability that a randomly selected person has diabetes, given that his test is positive?

`P(B|A) = (P(A \cap B))/(P(A)) = (0.095)/(0.1013) = 0.9378`

0.9378 = 93.78% probability that a randomly selected person has diabetes, given that his test is positive.