Question

If you divide any natural number n by 4, you get a remainder r.

Find r if n=13; 34; 43; 100. What is the domain of the
function? What is the range?

Answer 1

Given:

If you divide any natural number n by 4, you get a remainder r.

To find:

The values of r if
`n=13;34;43;100`. Also find the domain and range.

Solution:

It is given that any natural number n by 4, you get a remainder r.

`(n)/(4)=q+(r)/(4)`

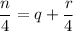
Where, n is a natural number, q is quotient, r is the remainder.

For
`n=13`,

`(13)/(4)=3+(1)/(4)`

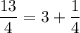
So,
`r=1`.

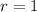For
`n=34`,

`(34)/(4)=8+(2)/(4)`

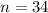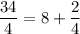
So,
`r=2`.

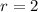For
`n=43`,

`(43)/(4)=10+(3)/(4)`

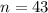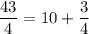
So,
`r=3`.

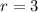For
`n=100`,

`(100)/(4)=25+(0)/(4)`

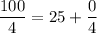
So,
`r=0`.

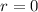Therefore, the required value are
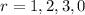`r=1,2,3,0` if
`n=13;34;43;100` respectively.

The domain of the function is
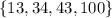`\{13,34,43,100\}` and the range of the function is
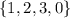`\{1,2,3,0\}`.