Given:
Radius of circle is 13 cm
Height of triangle is 5 cm
To find:
Value of 'a' and 'b'
Steps:
To find value of 'a', we will use Pythagoras theorem as the triangle is a right angle triangle,
A² + B² = C²
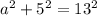
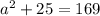
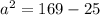
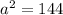
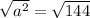
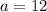
Now to find the value of 'b', i will use law of cosine,
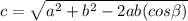
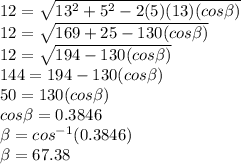
Therefore, the values of 'a' and 'b' is 12 and 67.38 respectively
Happy to help :)
If u need any help feel free to ask