Answer:
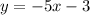
Explanation:
Hi there!
What we need to know:
Linear equations are typically organized in slope-intercept form:
 where m is the slope and b is the y-intercept (the value of y when x is 0).
where m is the slope and b is the y-intercept (the value of y when x is 0).
To solve for the equation of the line, we would need to:
- Find the point of intersection between the two given lines
- Use the point of intersection and the given point (-1,2) to solve for the slope of the line
- Use a point and the slope in
 to solve for the y-intercept
to solve for the y-intercept - Plug the slope and the y-intercept back into
 to achieve the final equation
to achieve the final equation
1) Find the point of intersection between the two given lines
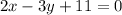
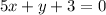
Isolate y in the second equation:
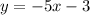
Plug y into the first equation:
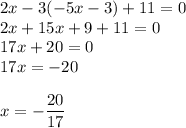
Plug x into the second equation to solve for y:
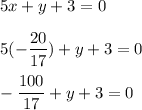
Isolate y:
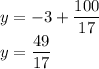
Therefore, the point of intersection between the two given lines is
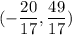 .
.
2) Determine the slope (m)
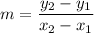 where two points that fall on the line are
where two points that fall on the line are
 and
and

Plug in the two points
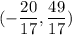 and (-1,2):
and (-1,2):

Therefore, the slope of the line is -5. Plug this into
 :
:

2) Determine the y-intercept (b)

Plug in the point (-1,2) and solve for b:
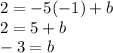
Therefore, the y-intercept is -3. Plug this back into
 :
:
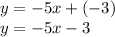
I hope this helps!