Answer:

Step-by-step explanation:
We are asked to find the new volume of a gas after a change in temperature. We will use Charles's Law, which states the volume of a gas is directly proportional to the temperature. The formula for this law is:
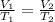
The gas was heated to 150 degrees Celsius and had a volume of 1587.4 liters.
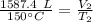
The temperature was 100 degrees Celsius, but the volume is unknown.
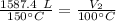
We are solving for the volume at 100 degrees Celsius, so we must isolate the variable V₂. It is being divided by 100°C and the inverse of division is multiplication. Multiply both sides of the equation by 100°C.
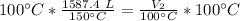
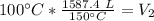
The units of degrees Celsius cancel.
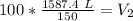

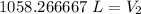
The original measurement of volume has 5 significant figures, so our answer must have the same. For the number we calculated, that is the tenth place. The 6 in the hundredth place to the right tells us to round to 2 up to a 3.
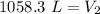
The volume of the gas at 100 degrees Celsius is approximately 1058.3 liters.