(C) You're given a probability mass function,
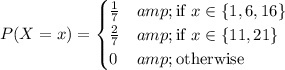
The mean is
![\displaystyle E[X] = \sum_x x\,P(X=x) = \frac{1*1}7 + \frac{1*6}7 + \frac{1*16}7 + \frac{2*11}7 + \frac{2*21}7 = \boxed{\frac{87}7} \approx 12.43](https://img.qammunity.org/2022/formulas/mathematics/college/r7ny6du3mpiiq75zxpn8oz0bns5frdwinw.png)
The variance is
![\displaystyle V[X] = \sum_x x^2\,P(X=x) = \frac{1^2}7 + \frac{6^2}7 + \frac{16^2}7 + \frac{2*11^2}7 + \frac{2*21^2}7 = \boxed{\frac{1417}7} \approx 202.43](https://img.qammunity.org/2022/formulas/mathematics/college/snsyf193x61ezglakzj1qvs0ry4zju3lkm.png)
The standard deviation is simply the square root of the variance:
![√(V[X]) = \boxed{\sqrt{\frac{1417}7}} \approx 14.23](https://img.qammunity.org/2022/formulas/mathematics/college/d1ow9jtdcqexp0slzh2fmwmxjpa89tmhr2.png)
(D) I'm not entirely sure what is being asked here, so I'm kinda guessing at the meaning. I think the question is saying there is a large set of 5000 test scores that are normally distributed with mean µ = 86 and standard deviation σ = 10.
Let X be the random variable representing these test scores. Then
(D.A)
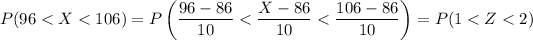
where Z follows the standard normal distribution with mean 0 and variance 1.
To find the remaining probability, you can use the empirical rule (68/95/99.7) which says
• approximately 68% of a normal distribution lies within 1 standard deviation of the mean; in other words,

• approximately 95% of the distribution lies within 2 standard deviations;
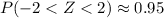
The normal distribution is also symmetric about its mean. Taking these facts together, we find
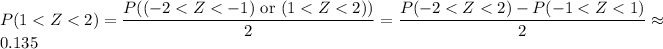
So roughly 13.5% of all test scores will fall between 96 and 106, and 13.5% of 5000 is 675. (The actual probability is closer 0.135905, and the projected test score count is closer to 679.)
(D.B) Any 50% of the distribution is still 50% of the distribution, so half of all the test scores would fall in this range. There would be 2500 test scores in that group.
(E) No choices given here...