Question

Please help me with this is so confusing

Answer 1

The expression for the height of the solid is:

`\displaystyle h = x^2+x-9`

Explanation:

Recall that the volume of a rectangular solid is given by:

`\displaystyle V = \ell wh`

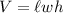
Where l is the length, w is the width, and h is the height.

We know that the volume is given by the polynomial:

`\displaystyle V = 3x^4-3x^3-33x^2+54x`

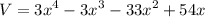
And that the length and width are given by, respectively:

`\displaystyle \ell = 3x \text{ and } w =x-2`

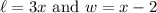
Substitute:

`\displaystyle 3x^4-3x^3-33x^2+54x=(3x)(x-2)h`

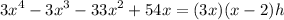
We can solve for h. First, divide both sides by 3x:

`\displaystyle (3x^4-3x^3-33x^2+54x)/(3x)=(x-2)h`

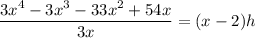
Divide each term:

`\displaystyle x^3-x^2-11x+18=(x-2)h`

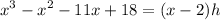
To solve for h, divide both sides by (x - 2):

`\displaystyle h = (x^3-x^2-11x+18)/(x-2)`

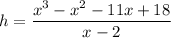
Since this is a polynomial divided by a binomial in the form of (x - a), we can use synthetic division, where a = 2. This is shown below. Therefore, the expression for the height of the solid is:

`\displaystyle h = x^2+x-9`