Answer:
0.3323
Step-by-step explanation:
GIven that:
Density of the metal = 10.6 g/cm^3
atomic weight = 176.8 g/mol
atmic radius = 0.130 nm
values of a and c = 0.570 nm and 0.341 nm respectively
For us to determine the atomic packing factor, we need to first determine the volume of all spheres (Vs) and the volume of unit cell (Vc).
However, the number of atoms in the unit cell (n) can be computed as:
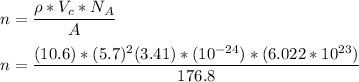
n = 4.0
Thus, the number of atoms in the unit cell is 4
∴
The atomic paking factor (APF) is calculated by using the formula:
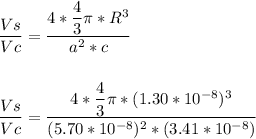
= 0.3323