Answer:
False
Explanation:
Before testing the hypothesis, we need to understand the central limit theorem and subtraction of normal variables.
Central Limit Theorem
The Central Limit Theorem establishes that, for a normally distributed random variable X, with mean
 and standard deviation
and standard deviation
 , the sampling distribution of the sample means with size n can be approximated to a normal distribution with mean
, the sampling distribution of the sample means with size n can be approximated to a normal distribution with mean
 and standard deviation
and standard deviation
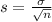 .
.
For a skewed variable, the Central Limit Theorem can also be applied, as long as n is at least 30.
For a proportion p in a sample of size n, the sampling distribution of the sample proportion will be approximately normal with mean
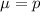 and standard deviation
and standard deviation
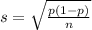
Subtraction between normal variables:
When two normal variables are subtracted, the mean is the difference of the means, while the standard deviation is the square root of the sum of the variances.
West University:
15 out of 100, so:
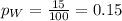
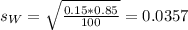
East University:
12 out of 100, so:


Test the difference in driving abilities at the two universities:
At the null hypothesis we test if there is no difference, that is, the subtraction of the proportions is 0, so:
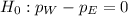
At the alternative hypothesis, we test if there is a difference, that is, if the subtraction of the proportions is different of 0. So
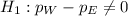
The test statistic is:
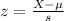
In which X is the sample mean,
 is the value tested at the null hypothesis, and s is the standard error.
is the value tested at the null hypothesis, and s is the standard error.
0 is tested at the null hypothesis:
This means that

From the two samples:
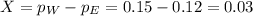
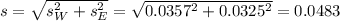
Value of the test statistic:
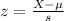
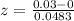
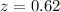
P-value of the test and decision:
The p-value of the test is the probability that the proportions differ by at least 0.03, which is P(|z| > 0.62), that is, 2 multiplied by the p-value of z = -0.62.
Looking at the z-table, z = -0.62 has a p-value of 0.2676.
2*0.2676 = 0.5352.
The p-value of the test is 0.5352 > 0.05, which means that the difference in driving is not statistically significant at the .05 significance level, and thus the answer is False.