Question

Given a triangle MTN, prove that

<m+<t+<n= 180° strictly
use m, T and N with other
Letters in your triangle.​

Answer 1

`\angle m + \angle t + \angle n = 180`

Explanation:

Required

Show that:

`\angle m + \angle t + \angle n = 180^o`

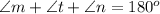
To make the proof easier, I've added a screenshot of the triangle.

We make use of alternate angles to complete the proof.

In the attached triangle, the two angles beside
`\angle m` are alternate to
`\angle t` and
`\angle n`

i.e.

`\angle 1 = \angle t`

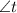`\angle 2 = \angle n`

Using angle on a straight line theorem, we have:

`\angle 1 + \angle m + \angle 2 = 180`

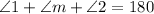
Substitute values for (1) and (2)

`\angle t + \angle m + \angle n = 180`

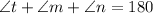
Rewrite as:

`\angle m + \angle t + \angle n = 180` -- proved