Answer:
On a coordinate plane, a hyperbola is shown. One curve opens up and to the right in quadrant 1, and the other curve opens down and to the left in quadrant 3. A vertical asymptote is at x = 1, and the horizontal asymptote is at y = 4
Explanation:
The given function is presented as follows;

From the given function, we have;
When x = 1, the denominator of the fraction,
 , which is (x - 1) = 0, and the function becomes,
, which is (x - 1) = 0, and the function becomes,
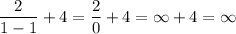 therefore, the function in undefined at x = 1, and the line x = 1 is a vertical asymptote
therefore, the function in undefined at x = 1, and the line x = 1 is a vertical asymptote
Also we have that in the given function, as x increases, the fraction
 tends to 0, therefore as x increases, we have;
tends to 0, therefore as x increases, we have;
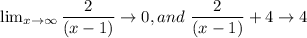
Therefore, as x increases, f(x) → 4, and 4 is a horizontal asymptote of the function, forming a curve that opens up and to the right in quadrant 1
When -∞ < x < 1, we also have that as x becomes more negative, f(x) → 4. When x = 0,
 . When x approaches 1 from the left, f(x) tends to -∞, forming a curve that opens down and to the left
. When x approaches 1 from the left, f(x) tends to -∞, forming a curve that opens down and to the left
Therefore, the correct option is on a coordinate plane, a hyperbola is shown. One curve opens up and to the right in quadrant 1, and the other curve opens down and to the left in quadrant 3. A vertical asymptote is at x = 1, and the horizontal asymptote is at y = 4.