Given:
The equation of a parabola is:
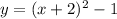
To find:
The coordinates of the vertex of the given equation.
Solution:
The vertex form of a quadratic function is:
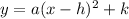 ...(i)
...(i)
Where, a is a constant and (h,k) is the vertex.
We have,
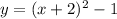 ...(ii)
...(ii)
On comparing (i) and (ii), we get
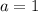
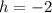
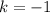
We know that the vertex of the parabola is (h,k).
Therefore, the vertex of the given equation is (-2,-1).