Answer:
1. a. 2
b. -½
c. y - 3 = -½(x - 8) => point-slope form
y = -½x + 7 => slope-intercept form
2. a. -1
b. 1
c. y - 5 = 1(x - 3) => point-slope form
y = x + 2 => slope-intercept form
Explanation:
1. (8, 3) and (10, 7):
a. The gradient for the line joining the points:
Gradient =
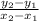
Let,
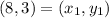
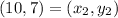
Plug in the values
Gradient =
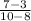
Gradient =

Gradient = 2
b. The gradient of the line perpendicular to this line = the negative reciprocal of 2
Negative reciprocal of 2 = -½
c. The equation of perpendicular line if it passes through the first point, (8, 3):
Equation of the perpendicular line in point-slope form can be expressed as y - b = m(x - a).
Where,
(a, b) = (8, 3)
Slope (m) = -½
Substitute (a, b) = (8, 3), and m = -½ into the point-slope equation, y - b = m(x - a).
Thus:
y - 3 = -½(x - 8) => point-slope form
We cam also express the equation of the perpendicular line in slope-intercept form by rewriting y - 3 = -½(x - 8) in the form of y = mx + b:
Thus:
y - 3 = -½(x - 8)
y - 3 = -½x + 4
y - 3 + 3 = -½x + 4 + 3
y = -½x + 7
2. (3, 5) and (4, 4):
a. The gradient for the line joining the points:
Gradient =
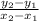
Let,
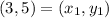

Plug in the values
Gradient =
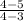
Gradient =
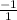
Gradient = -1
b. The gradient of the line perpendicular to this line = the negative reciprocal of -1
Negative reciprocal of -1 = 1
c. The equation of perpendicular line if it passes through the first point, (3, 5):
Equation of the perpendicular line in point-slope form can be expressed as y - b = m(x - a).
Where,
(a, b) = (3, 5)
Slope (m) = 1
Substitute (a, b) = (3, 5), and m = 1 into the point-slope equation, y - b = m(x - a).
Thus:
y - 5 = 1(x - 3) => point-slope form
We can also express the equation of the perpendicular line in slope-intercept form by rewriting y - 5 = 1(x - 3) in the form of y = mx + b:
Thus:
y - 5 = 1(x - 3)
y - 5 = x - 3
y - 5 + 5 = x - 3 + 5
y = x + 2