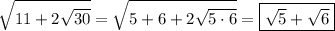2 Answers

Krupan · Answer 1 · 2022-01-18T05:53:19+0000

9514 1404 393

Answer:

√5 +√6

Explanation:

We know that the square of a binomial is ...

(a +b)^2 = a^2 +2ab +b^2

Then the square root of it is ...

a + b = √(a^2 +b^2 +2ab)

Using a=√x and b=√y, this is ...

√x +√y = √(x + y + 2√(xy))

__

For the given expression, we need to find x and y such that ...

xy = 30 and x+y = 11

Using x=5, y=6, we meet those requirements.

$\displaystyle \sqrt{11+2√(30)}=\sqrt{5+6+2√(5\cdot6)}=\boxed{√(5)+√(6)}$

Please log in or register to add a comment.