Question

Find the line integral with respect to arc length ∫C(9x+5y)ds, where C is the line segment in the xy-plane with endpoints P=(2,0) and Q=(0,7).

(a) Find a vector parametric equation r⃗ (t) for the line segment C so that points P and Q correspond to t=0 and t=1, respectively

(b) Rewrite integral using parametrization found in part a

(c) Evaluate the line integral with respect to arc length in part b

Answer 1

(a) You can parameterize C by the vector function

r(t) = (x(t), y(t) ) = P (1 - t ) + Q t = (2 - 2t, 7t )

where 0 ≤ t ≤ 1.

(b) From the above parameterization, we have

r'(t) = (-2, 7) ==> ||r'(t)|| = √((-2)² + 7²) = √53

Then

ds = √53 dt

and the line integral is

`\displaystyle\int_C(9x(t)+5y(t))\,\mathrm ds = \boxed{√(53)\int_0^1(17t+18)\,\mathrm dt}`

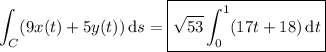
(c) The remaining integral is pretty simple,

`\displaystyle√(53)\int_0^1(17t+18)\,\mathrm dt = √(53)\left(\frac{17}2t^2+18t\right)\bigg|_(t=0)^(t=1) = \boxed{\frac{53^(3/2)}2}`