Question

A sample of butterflies contained 50% yellow-winged individuals and 50% black-winged individuals. In this species, wing color is determined by a single gene with two alleles, and the allele for black is dominant. Assume the sampled population of butterflies is in Hardy-Weinberg equilibrium. Also assume that the sample is random and large enough that the allele frequencies in the sample equal the allele frequencies in the population. What is the frequency of the allele for yellow wings in the population?

Answer 1

In the question, it is given that :

There are 50 percent yellow winged butterflies and 50 percent black winged butterflies.

The sampled population of the butterflies follows the Hardy-Weinberg equilibrium.

Therefore,

`p+q=1`

`p^2+pq+q^2=1`

`$q^2 = (50)/(100)$`

`$q^2 = 0.5$`

`$q=0.707$`

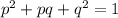
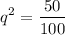
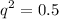
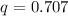
So,
`p=1-0.707`

`p = 0.293`

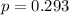
Therefore, the allele frequency for yellow winged butterflies will be q = 0.707