Question

An electron with an initial speed of 660,000 m/s is brought to rest by an electric field.

A) What was the potential difference that stopped the electron?
B) What was the initial kinetic energy of the electron, in electron volts?

Answer 1

A) ΔV = 1.237 V

B) K.E = 1.237 eV

Step-by-step explanation:

B)

The initial kinetic energy of the electron is given by the following formula:

`K.E = (1)/(2)mv^2\\\\`

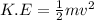
where,

K.E = Kinetic Energy of electron = ?

m = mass of elctron = 9.1 x 10⁻³¹ kg

v = speed of electron = 660000 m/s

Therefore,

`K.E = (1)/(2)(9.1\ x\ 10^(-31)\ kg)(660000\ m/s)^2`

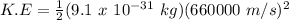
K.E = 1.98 x 10⁻¹⁹ J

K.E = (1.98 x 10⁻¹⁹ J)(
`(1\ eV)/(1.6\ x\ 10^(-19)\ J)`)

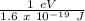K.E = 1.237 eV

A)

The energy applied by the potential difference must be equal to the kinetic energy of the electron, in order to stop it:

`e\Delta V = K.E\\\\\Delta V = (K.E)/(e)`

where,

e = charge on electron = 1.6 x 10⁻¹⁹ C

Therefore,

`\Delta V = (1.98\ x\ 10^(-19)\ J)/(1.6\ x\ 10^(-19)\ C)`

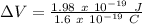
ΔV = 1.237 V