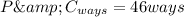Answer:
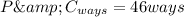
Explanation:
From the question we are told that:
Sample size

Generally the pitcher or catcher field position can be filled in
23 way respectively
Where
No. ways for to fill Pitcher

No. ways for to fill Catcher
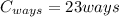
Therefore
Applying counting Principles
No. ways to fill both