Step-by-step explanation:
Given that,
Five ideal-gas molecules chosen at random are found to have speeds of 500, 600, 700, 800, and 900 m/s.
The rms speed for this collection is as follows :
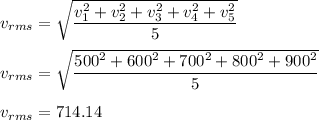
The average speed of these molecules is :

So, the rms speed is 714.14 m/s abd the average speed is 700 m/s.