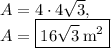Question

Plzz helpppppp222!!!!!!!!!!!!

Answer 1

Explanation:

The rectangle has 4 corners of 90 degrees.

Here a rectangle is divided into two right triangles. In right-angled triangles, the opposite side at a 30-degree angle is half a chord...I replace x instead of length.. So x / 2 = 4--->x:8

The perimeter of a rectangle is equal to (length + width) × 2--->(8+4)×2=24m^2

Answer 2

`\text{(D) }16√(3)\:\mathrm{m^2}`

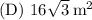
Explanation:

In all 30-60-90 triangles, the sides are in ratio
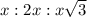`x:2x:x√(3)`, where
`x` is the side opposite to the 30 degree angle and
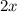`2x` is the hypotenuse of the triangle.

The rectangle shown forms two 30-60-90 triangles. The side labelled 4 meters is opposite to the 30 degree angle. Therefore, the side on top must be
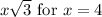`x√(3)\text{ for }x=4`. Let the length of the top base be
`w`:

`w=4√(3)`

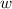The area of a rectangle with length
`l` and width
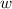`w` is given by
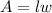`A=lw`. Thus, the area of the rectangle is:

`A=4\cdot 4√(3),\\A=\boxed{16√(3)\:\mathrm{m^2}}`