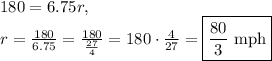Answer:

Explanation:
We can use the formula
 (distance = rate * time) to solve this problem.
(distance = rate * time) to solve this problem.
If the motorist is travelling 90 miles to and back on a trip, he has travelled
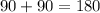 miles total. This represents
miles total. This represents
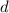 in our formula.
in our formula.
Now we need to find the total time.
On the first trip, it's given that the motorist travels at a rate of 20 mph. Therefore, the time this trip took to travel 90 miles is:
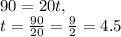 hours
hours
On the second trip back, he travels the same distance (90 miles) at a rate of 40 mph. Therefore, the time the trip took is:
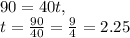 hours
hours
Therefore, the total time is
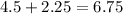 hours.
hours.
Now can calculate the average speed of the entire trip: